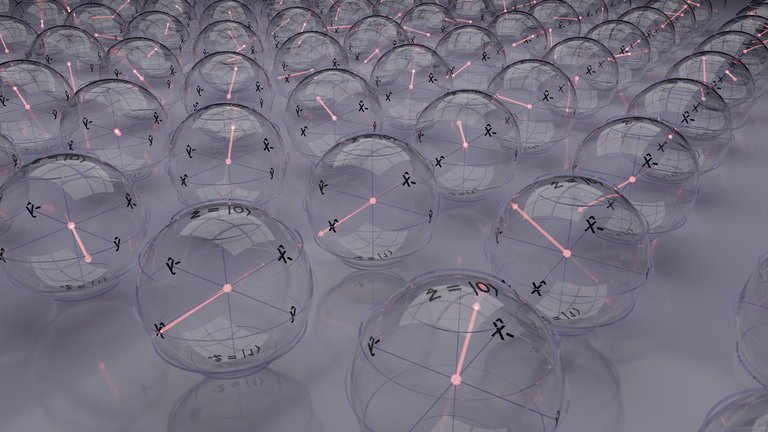
The Bloch sphere is a unit 2-sphere which is a topological object homeomorphic to a 3-dimensional Euclidean sphere. The antipodal points of a 2-sphere correspond to a pair of mutually orthogonal state vectors. The north and south poles of the Bloch sphere are typically chosen to correspond to the standard basis vectors, which in turn might correspond e.g. to the spin-up and spin-down states of an electron. This choice is arbitrary, however. The points on the surface of the sphere correspond to the pure states of the system, whereas the interior points correspond to the mixed states. [2][3]
3D installation
When we apply a quantum gate to a qubit, we change it in some way. This is similar to how classical logic gates modify bits. For instance, the NOT gate in classical computing outputs a 1 if a 0 is inputted and vice versa. These operations can be visualized as rotations of a state vector on the Bloch sphere. For this reason this tool is a powerful visualization of the basic operations in quantum computing.
By transforming this abstract concept into a 3D installation we aim at showing the basic of quantum computing to the largest possible non-specialist audience.
- Bloch, Felix (Oct 1946). "Nuclear induction". Phys. Rev. 70 (7–8): 460–474. Bibcode:1946PhRv...70..460B (http s://ui.adsabs.harvard.edu/abs/1946PhRv...70..460B). doi:10.1103/physrev.70.460 (https://doi.org/10.1103%2Fph ysrev.70.460).
- Nielsen, Michael A.; Chuang, Isaac L. (2004). Quantum Computation and Quantum Information. Cambridge University Press. ISBN 978-0-521-63503-5.
- https://www.quantiki.org/wiki/bloch-sphere